I had asked the five-year-old children to suggest ‘frog jumps’ on the class number line. To model subtraction the frog, ‘Freddie’, is placed on the line and then jumps back the number subtracted. Giannis has suggested that Freddie starts on the number 1.
| MTF | [Places frog above the number 1 on class line] He didn’t go very far! [Laughs] Now let’s see what Giannis is going to say [pause] |
| Giannis | [Smiles] Two! |
| MTF | He jumps back [pause] Two?! [Puts out two fingers] Let’s see what happens if Freddie jumps back two. One, two! [Moves frog back one jump, then another. Mimics the frog falling half way to the floor] |
| Child 1 | Woo! |
| David | Aaah! |
| MTF | [Moving the frog toward the floor] What happens to Freddie? |
| Sven | He fell off! [Smiles] |
| Children | [Smile] He falls off / he fell off. |
| Dragan | [Smiles] He jumped in the water. |
This vignette is taken from a series of lessons I carried out with a group of young children in Malta as part of a teaching/research experience. The frog jumps—which were key to the children’s learning about subtraction—are the focus of this article.
As a teacher-educator, I teach elementary mathematics education to pre-service teachers, and frequently have contact with in-service teachers. One of my main areas of interest is language, and in my discussions with practitioners, I encourage them to give explicit attention to mathematical language. I wished to try out this recommendation for myself through a teaching/research experience in order to ‘practise what I preached’ and to be in a better position to reflect on this aspect of mathematics education. One of the classes I taught for this purpose included Giannis and the other children featured in the vignette above.
Elsewhere (Farrugia, 2017) I focus on the children’s appropriation of aspects of the mathematics register in relation to subtraction as separation or ‘take away’. Here I consider subtraction on the number line, or ordinal subtraction, and focus on the integration of the various resources used, which I consider from a semiotic perspective. I offer an example of the application of semiotic theory to a practical teaching situation. Following this, I hone in more specifically on the function of a gesture mimicking a jumping frog. Using ‘everyday’ items is a common approach to addressing mathematical ideas with young children, and therefore I believe it is useful to study the role of gesture as they are used in conjunction with such items.
The research context
Maltese is the home language of most of the population, but English plays an important role in many spheres of life and is the academic language of various subjects, including mathematics. Generally, during mathematics lessons it is common for teachers and students to mix Maltese and English for verbal communication whereas all written work is in English.
However, from my professional experience, I can say that when faced with a class of mixed nationalities, teachers will tend to increase their use of English. Although English may not be a child’s home language, it is more likely that they would know—or to be supported by family members to learn—some English rather than Maltese. The class that participated in this study included a high number of such children. It comprised 22 five-year-olds representing 13 nationalities. For example, the children named in the vignette above include Giannis (Greek), David (Maltese), Sven (Finnish/Kenyan), and Dragan (Serbian/Maltese).
The class teacher, Ms Jenny, reported that all the children understood English, although their oral fluency varied from “doesn’t speak English” to “very fluent”. On the other hand, she described six children as having no or limited understanding of Maltese. The mix of nationalities in the class prompted Ms Jenny to use English as a lingua franca for mathematics, using Maltese occasionally with Maltese students. During my own lessons, I too used English as the main language of instruction, with occasional Maltese.
After some informal observations, I substituted for Ms Jenny for eight mathematics lessons. The lessons were video-recorded and the children showed up on the camera if they, and their parents, consented. ‘Subtraction’ was a new school topic for the children. They were already familiar with the number line and the symbols + and = from previous lessons on addition carried out with Ms Jenny. I introduced the minus sign during our activities on subtraction as separation or ‘take away’. Hence, the children were already familiar with the symbol when we worked on ordinal subtraction, the focus of the present article.
My main interest while planning the lessons was English mathematics terminology in order to support the children in expressing concepts and ideas (Lee, 2006). Thus, my planning included ‘lining up’ mathematics and language objectives as recommended by Gibbons (2015). My teaching approach included stressing verbal expressions and offering sentence frames with which the children could work in pairs or contribute to a class discussion. This approach to teaching mathematical language is encouraged by researchers such as Bresser, Melanese and Sphar (2009), who consider it particularly helpful with second language learners.
A semiotic interpretation of the teaching/learning process
As I planned the approach and activities that I was going to use in class, I was influenced by social semiotics. Social semiotics is a theoretical perspective that is concerned with how people construct systems of meanings through social interaction (Chapman, 2003), and hence it provides a relevant perspective for interpreting mathematics teaching and learning. By explaining the teaching/learning process using a semiotic perspective, I offer an illustration of an application of the theory. In particular, I draw on the work carried out by Maria G. Bartolini Bussi, Ferdinando Arzarello and their colleagues.
Bartolini Bussi and her colleagues have developed a semiotic approach to teaching and learning based on a Vygotskian perspective, and centres on the artefact. Following Rabardel (1995), Bartolini Bussi and Mariotti (2008) distinguish between a material object per se, originally designed with some purpose in mind, and the use to which the object is put in the classroom. In the latter case the object acts as an instrument which is a “mixed entity […] born of both the subject and the object” (Rabardel & Samurçay, 2001, p.20). According to Bartolini Bussi and Mariotti (2008) an artefact can be referred to as a tool of semiotic mediation as long as it is (or is conceived to be) intentionally used by the teacher to mediate some mathematical content. Thus, the teacher takes the role of cultural mediator (Mariotti, 2009). This role does not refer simply to the use of a tool or artefact to accomplish a task “but rather to the fact that new meanings, related to the actual use of the tool, may be generated, and evolve, under the guidance of the expert ( i.e., the teacher)” (Bartolini Bussi & Mariotti, 2008, p. 754). Hence, an artefact has ‘semiotic potential’ that may be constructed and exploited (Bartolini Bussi, Corni, Mariani & Falcade, 2012, p. 6). This potential is the double semiotic link between the artefact and, on one hand the immediate (situated) task to be accomplished and, on the other hand, mathematical meanings evoked by its use. Arzarello, Paola, Robutti and Sabena (2009) take a similar view, pointing out that signs may be personal (e.g., an idiosyncratic gesture carried out by a student) or institutional (e.g., algebraic notation). A teacher’s aim is therefore to support student appropriation of culturally shared, or ‘institutional’ meaning of signs.
For my lessons on ordinal subtraction, the key resource, or artefact, used was a picture of a green frog named Freddie. This item was to act as an instrument, since I intentionally planned to use it to address some mathematical content. There were two versions of the frog. The large one was attached to a broomstick and was generally used by myself. It was made to ‘jump’ along the classroom number line, consisting of large separate number cards attached with clothes pegs to a string that ran across the room. The children had similar small frogs which were used in conjunction with small laminated (continuous) number lines on their tables. These items are shown in Figures 1 and 2.

Figure 1. The class number line and large frog on a broomstick.
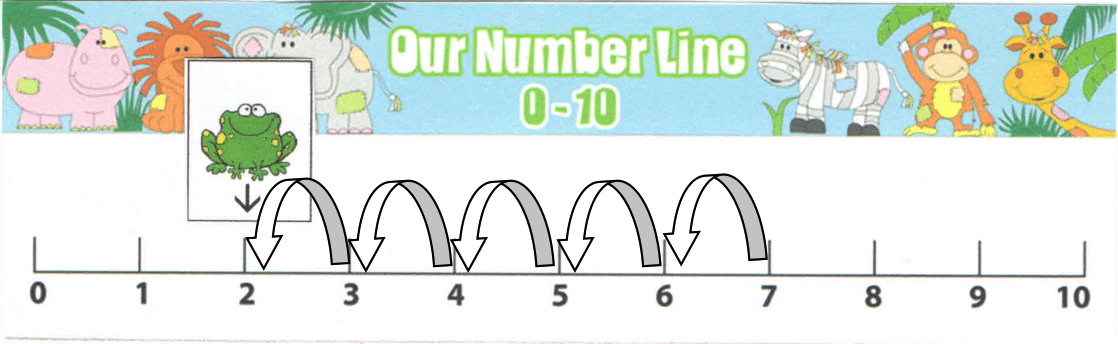
Figure 2. Student laminated number line and small frog.
Occasionally, and especially when I first handed out the small frogs, the children carried out their own personal actions with this item. For example, David held the frog up high above his head, then dropped it to the table, or onto Dragan’s head; Dragan slid the frog along his desk, telling me that he had named his frog ‘Foxy’. Mohammed and Shania attached their frogs to their index fingers with adhesive putty and wagged the fingers at each other, as though their frogs were chatting. Bartolini Bussi and Mariotti (2008) refer to these personal actions as artefact signs since they arise from the individual or paired activity related directly with the object. These signs can include writing, drawing, language and gestures and their meanings are personal.
While I did not stop the children from ‘playing’, or rather, carrying out their own personalised actions with the artefact, it was necessary to get them focused on the jumping action in order to approach the mathematics I had in mind. My ultimate aim was for the children to access mathematical signs which, as explained by Bartolini Bussi and Mariotti, relate to the mathematical meanings as shared by the wider community. Thus, I channeled the children to use the artefact in a particular way, in order to enable them to set up a link between the item, language, symbols, and graphics. Arzarello (2006) considers the combined use of various resources as a ‘semiotic bundle’. He defines a semiotic bundle as a collection of sets of signs that are used in relation to an artefact (speech, writing, gestures, gazes, drawing and others) together with a set of relationships between the sets. In my case, the semiotic sets used in conjunction with the artefact were:
- Spoken language, including the expressions ‘count back’, ‘jump back’/‘minus’, ‘equals’; written language: ‘count back’
- Symbols: numerals 0–10, –, =
- Graphics: representations of the number line on worksheets
- Gestures: the key gesture of causing a frog to jump on the number line (large / small frogs), and using finger movements to indicate a backward direction on the line. (Pointing and other gestures are not given prominence in this paper; similarly, gazes are mentioned briefly, but not examined in detail.)
The semiotic bundle initially included the physical artefact (frog), spoken language, the numerals (symbols), gestures and gazes. An example is shown in Table 1, an annotated transcript involving Andrea, who is suggesting an example, and the rest of the children, who are listening attentively. In the transcript, the components of the bundle are separated in what Arzarello (2006) calls a synchronic analysis of the bundle, wherein the simultaneous components of the unitary system are distinguished.
| Participants | Language | Gestures/gazes | Symbols |
| Andrea | Freddie is on number ten. | Numerals 1-10 | |
| MTF | Very good, she said it so clearly. | Places frog above the ten. | 10 |
| Andrea | Moves nine. | ||
| MTF | I’m going to move him nine. Come on Freddie, jump! | Looks at and ‘addresses’ the frog which is being held above the number 10. | 10 |
| MTF | One, two, three, four, five, six, seven, eight, nine. | Moves frog in jumps backwards along the number line. | Numerals 1–9 |
| Children | two, three, four, five, six, seven, eight, nine. | Looking attentively at teacher’s gestures and number line. | Numerals 1–9 |
| MTF | Andrea, ask your friends the question [pause] What number [pause] ? | ||
| Andrea | What number is Freddie on? | Looks around her. | 1 |
| Children | One! | Look at frog above number 1. | 1 |
Table 1. Initial components of the semiotic bundle
The interaction was a ‘collective production’ of the semiotic sets (Bartolini Bussi and Mariotti, 2008). In my teaching design, collective production tended to precede the individual production of signs since starting with a class discussion allowed me to introduce the necessary language, including sentence frames, that I wished to encourage.
Arzarello (2006) argues that learning occurs through a dynamically developing bundle, which enlarges through genetic conversions. ‘Enlargement’ of a bundle refers to an increase in the number of active sets within the bundle and an increase in the number of relationships (and transformations) between the different semiotic sets. Hence, a bundle can also be analysed diachronically, that is over time, as lessons progress (Arzarello, 2006). Over the course of my lessons the semiotic bundle was enlarged through the inclusion of graphics and written language. These were brought into play through the children’s engagement with their small number lines and worksheets. The semiotic bundle was further enlarged with the introduction of symbols. For example, as one child instructed the frog to move, another child would suggest the institutional signs that could be used to represent the movement (e.g., 6 – 4 = 2) and thus to express ordinal subtraction. The gradual genetic conversions that I targeted in order to enable a move from the context of a jumping frog to institutionalised meaning of symbols symbolisation are shown in Figure 3. The first row refers to oral expression related to the artefact; the second row refers to written symbols, text and non-institutional symbols; the third row refers to institutional signs.

Figure 3. The introduction of institutional symbolisation.
I now focus more specifically on one element of the bundle, namely, the gesture of moving or mimicking the jumping frog.
A gesture as a pivot sign
Bartolini Bussi and Mariotti (2008) define pivot signs as ones that enable the passage from artefact to mathematical context. These signs link the artefact with the mathematical context by way of their relation to both. Through the analysis of my data I noted how a gesture can serve as a pivot sign.
The importance of gestures in the development of mathematical ideas has been given attention in recent research. Indeed, drawing on the work of David McNeill (for McNeill see, for example, 1992, 2005), Arzarello et al. (2009) and Arzarello, Robutti and Thomas (2015) give particular attention to the gesture as a component of the semiotic bundle in the context of high school students working on graphs. In a similar vein, and in relation to high-school students working on the parabola, continued fractions and induction. Krause (2016) gives details of how gestures served to prepare, support and realise epistemic actions, thus serving an “epistemic function” (p. 246). She notes the use of particular gestures that became situationally conventionalised through recurrent use, and thus became associated with a mathematical idea or object as it developed.
In my study the key gesture was one that moved the frog, or mimicked this movement. The initial gesture used was that of moving the frog along the class number line and along the children’s individual number lines. Thus, as noted by Krause (2016), a situationally conventionalised gesture became associated with a mathematical idea. However, by the third lesson, few children bothered to use the small frog when using their number lines or when completing their worksheet. Some children told me that they did not need the frog as I handed the little pictures out, while other children simply left the frog unused on their table. Instead, these children carried out subtractions on the number line by moving their index finger backwards along the line in arch-like movements. Thus, the gesture of moving the frog was modified to a related finger movement. This gesture appeared to serve as a pivot sign (Bartolini Bussi & Mariotti, 2008) which enabled the passage from artefact to mathematical context, namely subtraction with numbers up to 10. After a while, some children appeared to work out answers to subtractions by only looking at the number line printed on their worksheet, giving me the impression that they were ‘performing’ the gesture mentally.
The ordinal interpretation of subtraction is based on the grounding metaphor arithmetic is motion as described by Lakoff and Núñez (1997, pp. 34-35). The metaphor is ‘grounding’ since it grounds mathematical ideas in everyday experience, in this case moving through space. In accordance with this metaphor, numbers are locations on a path, zero is the origin, operations are acts of moving on that path, and subtraction is taking steps a given distances to the left (or backwards). Since the gestures of moving the frog and moving a finger are linked to the grounding metaphor, I refer to them as grounding gestures. I suggest that it is at the stage of mental manipulation that projection of the motion-metaphor to the domain of mathematics is achieved. The stages are summarised in Table 2.
| Gesture | Relation to mathematics |
|---|---|
| Causing the frog to jump along the number line. | Action on artefact—grounding gesture shows an everyday experience (frog movement through space ) |
| Moving finger along the number line. | Modified action; pivot sign |
| ‘Mental’ movement along the number line through gaze. | No physical gesture; the focus is number relationships based on the metaphor arithmetic is motion (Lakoff & Núñez, 1997). |
Table 2. From grounding gestures to the mathematical domain.
The extension of the number line
The five-year-old children were, of course, too young to appreciate an extension of the number line to negative numbers; according to Ms Jenny, a few of them were still consolidating their knowledge of the counting numbers, and they had only recently been introduced to the number line during the topic addition. For the purpose of my lessons, the 0 was assumed to be the end of the line. However, in the course of the class discussions, the idea of left-of-zero cropped up and I believe this awareness can be considered to be an early first step to an appreciation of the potential extension of the number line. Indeed, Lakoff and Núñez (1997) consider the motion metaphor to offer to “easiest natural extension […] to negative numbers” (p. 39). The vignette I presented at the beginning of this article formed part of the discussion of the ‘end of the line’. I now return to the vignette, giving details of what came before and after, thus rendering its significance to the learning about subtraction more evident.
In the lesson before, Shania suggested the frog starts on 1, then jumps back 2. The other children called out in chorus “zero!” while I said that Freddie had fallen off the line, carrying out a falling gesture to the floor using the large frog. I did not consider it necessary to pursue the point further; considering their age I accepted their chorus answer of “zero” and moved on. The next day I asked the children to recapitulate the previous day’s work. Giannis had put up his hand eagerly, with a smug smile on his face. At the time, and again on revisiting the video, it is evident that Giannis had something ‘up his sleeve’. The unnamed children are off camera and not identified.
| MTF | [On Giannis’ request, MTF places frog above the number 1 on class line] He didn’t go very far! [Laughs] Now let’s see what Giannis is going to say [pause] |
| Giannis | [Smiles] Two! |
| MTF | He jumps back [pause] Two?! [Puts out two fingers] Let’s see what happens if Freddie jumps back two. One, two! [Moves frog back one jump, then another. Mimics the frog falling half way to the floor] |
| Child 1 | Woo! |
| David | Aaah! |
| MTF | [Moving the frog toward the floor] What happens to Freddie? |
| Sven | He fell off! [Smiles] |
| Children | [Smile] He falls off / he fell off. |
| Dragan | [Smiles] He jumped in the water. |
| MTF | He falls off the line! Giannis, would you like to change it, so that Freddie DOESN’T fall off the line? |
| Giannis | [Smiles] Three! |
| MTF | Three?! Let’s see what happens. |
| David | No, because he will fall down [Sounds concerned] |
| MTF | One, two [pause] |
| David | Aahh! |
| MTF | [pause] three! [Moves frog to zero then down toward the floor] He’s gone right to the bottom of the pond! [Mimics the frog falling almost to the floor] |
| Children | [Smile and squeal] |
| MTF | I need a number so that he won’t fall off! |
| Children | One / two. |
| MTF | When he jumped two, he fell off. I wonder who can tell me [pause] |
| Children | [Put their hands up eagerly] One, one! |
| MTF | I think that Giannis KNEW that it was one, but he was playing a trick on Freddie! I think he wanted him to fall off. |
| Child 2 | Even I! |
At the time, it appeared to me that Giannis had what Barnes (2000) calls a ‘magical moment in mathematics’, which she describes as a moment of sudden realisation of new understanding, accompanied by a positive emotion such as delight or satisfaction. Perhaps Giannis had held onto this thought from the previous day when I had commented that the frog had fallen off the line, or perhaps he came to ‘see’ it there and then. I am not in a position to say. The idea may have been prompted by what was the favourite example of many of the children’s, namely, instances when the frog landed on zero ( e.g., 5 – 5 = 0). Interestingly, Giannis’ insight appeared to be quickly taken up by other children. As evidence for this, I consider David’s concern that “he will fall down”, the children’s laughter and squealing, Child 2’s claim that he too wished to drop the frog off the line, and the children’s ability to suggest numbers such that the frog would not fall off the line. This illustrates Dooley’s (2007) point that whole-class settings can be the site for the construction of collective ‘aha’ moments. As Dooley points out, it is difficult to analyse to what extent all members of the large group have understood, and I can only draw conclusions for some of the children based on their classroom input and interview data as illustrated in the following excerpt taken from Mohammed’s interview. Here we were discussing subtraction with respect to the small laminated number line.
| Mohammed | [Places the frog on 10] I’m going to do something funny! Jump ten, no eleven. [Counts while moving the frog back] […] nine, ten, eleven. BOOM! [Moves frog off the line] |
| MTF | What happens when you move eleven? |
| Mohammed | It’s fall. |
| MTF | Can you give me an example when he does NOT fall off the line? |
| Mohammed | Aha [yes]. Move three. |
This excerpt shows that Mohammed was able to distinguish between numbers that would take the frog off the line and ones that would not, and that that the classroom experiences had helped him become aware of the ‘distance’ and the ‘zero is the origin’ elements suggested by the arithmetic as motion metaphor.
Conclusion
My original aim in undertaking this teaching/research experience was to explore the explicit teaching of mathematical language. Thus during the planning phase my attention was more on the language elements of the semiotic sets to be used, rather than on gesture. However, on analysis of the videos I was prompted to reflect in more detail on the key gesture that represented movement on the number line. Due to the close integration of various elements of the semiotic bundle, it is not possible to tease out the influence of each element on children’s learning. However, the children’s active engagement with the jumping frog and related actions suggests that the gesture was an important factor in their learning of ordinal subtraction.
The power of gestures is well recognised in general mathematics education, so possibly the jumping gesture would be useful in any classroom of young children, even a monolingual group. Bresser, Melanese and Sphar (2009) stress however that gestures are important to use with second language learners. Possibly, since English was the second language of many of the children in the class and the school topic subtraction was new to them, the gesture may have been particularly helpful. I suggest that the action of causing the frog to jump may have offered a (presumably universally understood) element with which to interpret the new English terminology like jump back, minus, and so on, and the new symbolisation e.g., 7 – 2 = 5. The meaning potential of the artefact, the frog, was further extended through the suggestion of it falling off the line.
The gesture was originally related directly to the artefact but was modified by the children into arch-like finger movements as they moved along their number lines. This movement appeared to act as a pivot sign, linking the frog context to the mathematical domain. Indeed, over the lessons, a number of children stopped using the frog and their fingers, but seemed to perform the gesture mentally.
I consider the gestures of moving the frog and finger to be grounding gestures since they are related to the grounding metaphor arithmetic is motion (Lakoff & Núñez, 1997). Similar gestures would be applicable for carrying out other operations in the number line. For example, by taking steps a given distance to the right (addition) or by repeatedly segmenting a path of given length into as many smaller paths of a given length (division, as described by Lakoff & Núñez). Movement is also used when carrying out operations on a 100-grid, which is another commonly used resource in elementary school. It may be interesting to study movement gestures associated with this resource. Other metaphors for arithmetic given by Lakoff and Núñez are arithmetic is object collection and arithmetic is object construction. My teaching of subtraction as ‘take away’, carried out in the days prior to ordinal subtraction, would fall under the former metaphor. Here “subtraction is taking smaller collections from larger collections to form other collections” (p. 35). A next research step for me could be to re-visit the ‘take away’ lessons and analyse the gestures used in these lessons to describe their relation with the underlying metaphor.
More generally, more research can be done to explore the role of gesture in semiotic bundles (Arzarello, 2006) utilised in elementary school classrooms. It is common practice for teachers of young children to use ‘everyday’ items as a starting point for mathematical ideas. It would be interesting to explore if, and how, gestures serve as pivot signs to help the children—whether first or second language learners—move from the immediate context to the mathematical domain.
References
Arzarello, R. (2006) Semiosis as a multimodal process. RELIME: Revista Latino Americana de Investigación en Matemática Educativa, 9 (1), 267-299.
Arzarello, F., Paola, D., Robutti, O. & Sabena, C. (2009) Gestures as semiotic resources in the mathematics classroom. Educational Studies in Mathematics, 70, 97-109. DOI: 10.1007/s10649-008-9163-z
Arzarello, F., Robutti, O. & Thomas, M. (2015) Growth point and gestures: looking inside mathematical meaning. Education Studies in Mathematics, 90(1), 9-37. DOI: 10.1007/s10649-015-9611-5
Barnes, M. (2000) ‘Magical’ moments in mathematics: insights into the process of coming to know. For the Learning of Mathematics, 20(1), 33–43.
Bartolini Bussi, M.G., Corni, F., Mariani, C. & Falcade, R. (2012) Semiotic mediation in mathematics and physics classrooms: artifacts and signs after a Vygotskian approach. Electronic Journal of Science Education, 16(3), 1–28. http://ejse.southwestern.edu.
Bartolini Bussi, M.G. & Mariotti, M.A. (2008) Semiotic mediation in the mathematics classroom: artifacts and signs after a Vygotskian perspective. In English, L., Bartolini Bussi, M.G., Jones, G., Lesh, G. & Tirosh, D. (Eds.) Handbook of International Research in Mathematics Education (Second Edition Revised), pp. 746–783. Mahwah, NJ: Lawrence Erlbaum.
Bresser, R., Melanese, K. & Sphar, C. (2009) Supporting English Language Learners in Math Class, grades K–2. Sausalito, CA: Math Solutions.
Chapman, A. (2003) A social semiotic of language and learning in school mathematics. In Anderson, M., Sáenz-Ludlow, A., Zellweger, S. & Cifarelli, V.V. (Eds.), Educational Perspectives on Mathematics as Semiosis: From Thinking to Interpreting to Knowing , pp. 129-148. New York: Legas.
Dooley, T. (2007) How the ‘stumbling of ideas across each other’ facilitates insight in primary mathematics. In Close, S., Corcoran, D. & Dooley, T. (Eds.). Proceedings of the Second National Conference of Research in Mathematics Education , pp. 44–55. Dublin: St. Patrick’s College.
Farrugia, M.T. (2017) Young children’s appropriation of mathematical discourse: learning subtraction in a plurilingual classroom. Paper presented at the 10th Congress of the European Society for Research in Mathematics Education (CERME10), Dublin City University, 1–5, February 2017.
Gibbons, P. (2015) Scaffolding language, scaffolding learning (2nd edition). Portsmouth, NH: Heinemann.
Krause, C.M. (2016) The Mathematics in our Hands: How Gestures Contribute to Constructing Mathematical Knowledge . Wiesbaden: Springer Spektrum.
Lakoff, G. and Núñez, R.E. (1997) The metaphorical structure of mathematics: sketching our cognitive foundations for a mind-based mathematics. In English, L.D. (Ed.), Mathematical Reasoning: Analogies, metaphors and images, pp. 21–89. Mahwah, NJ: Lawrence Erlbaum Associates.
Lee, C. (2006) Language for Learning Mathematics: Assessment for Learning in Practice . Maidenhead: Open University Press.
Mariotti, M.A. (2009) Artifacts and signs after a Vygotskian perspective: the role of the teacher. ZDM Mathematics Education, 41, 427–440. DOI:10.1007/s11858-009-0199-z
McNeill, D. (1992) Hand and Mind: What Gestures Reveal about Thought. Chicago: University of Chicago Press.
McNeill, D. (2005) Gesture and Thought. Chicago: University of Chicago Press.
Radardel, P. (1995) Les Hommes et les Technologies—Approche Cognitive des Instruments Contemporains . Paris: A. Colin.
Radardel, P. and Samuçay, R. (2001) From artifact to instrumented-mediated learning: new challenges to research on learning . International symposium organized by the Center for Activity Theory and Development Work Research, University of Helsinki, March 21–23.